Probability
Extension Problems
(a) What is the probability of reaching the goal starting at position A?
(b) What is the probability of reaching the goal starting at position B?
(c) What is the probability of reaching the goal starting at position C?
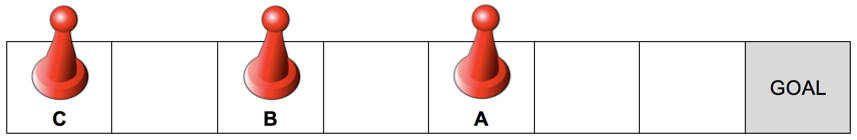
*Give your answers as fractions in simplest terms.
The following is true of four of the people:
- Allen is older than Bob.
- Bob is older than Carrie.
- Bob is older than Dave.
Assume that for any particular age, each person has the same chance to be that age.
*Give your answer as a fraction in simplest terms.
When two fair six-sided die are rolled, what is the probability that the sum of the square of the numbers rolled is a multiple of 4?
*Give your answer as a fraction in simplest form
There are $n$ cards numbered $1,2,\ldots \;n$ where $n\ge 3$.
Three cards are drawn one after another without replacement. Let the numbers on these cards be $a, b,$ and $c$ repsectively. Find the probability that $a \lt b \lt c$.
*Give your answer as a fraction in simplest form
Weather forecaster $A$ is correct about their predictions $70\%$ of the time and
weather forecaster $B$ is correct about their predictions $60\%$ of the time. These probabilities are independent of each other.
$A$ claims “it will rain tomorrow” and $B$ claims “it will not rain tomorrow”.
What is the probability that it rains tomorrow?
*Give your answer as a fraction in simplest form
Deck A contains five cards numbered from $0$ to $4$.
Deck B contains ten cards numbered from $0$ to $9$.
One card from deck A and two cards from deck B are drawn without replacement.
Find the probability that the product of the numbers on these three cards equals zero.
*Give your answer as a fraction in simplest form
A box contains 9 coins.
8 of the coins are fair coins with one side heads and one side tails. 1 of the coins is unfair and has heads on both sides.
A coin is randomly chosen and flipped 4 times, getting all heads. It is unknown whether the coin was a fair or the unfair coin.
If this coin is flipped again, find the probability that it will be heads.
*Give your answer as a fraction in simplest form
A bag contains $2$ red balls and $1$ blue ball. A ball is randomly pulled out of the bag.
If it is red, it is replaced with a blue ball and put back in the bag.
If it is blue, it is replaced with a red ball and put back in the bag.
After repeating this four times, what is the probability that the $3$ balls in the bag are all blue?
*Give your answer as a fraction in simplest form
There is a box full of gold, silver and bronze coins. $3$ coins are chosen at random.
There is a bag full of gold and silver rings. $2$ rings are chosen at random.
If it is known that exactly $3$ of the $5$ items chosen are gold, find the probability that exactly two types of coins were chosen.
*Give your answer as a fraction in simplest form